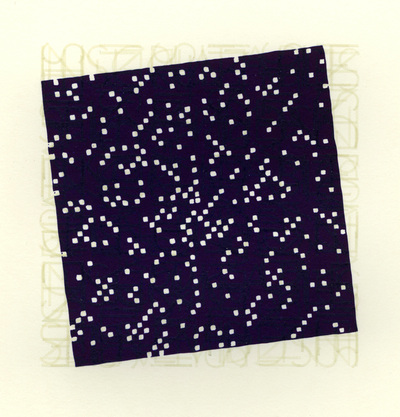
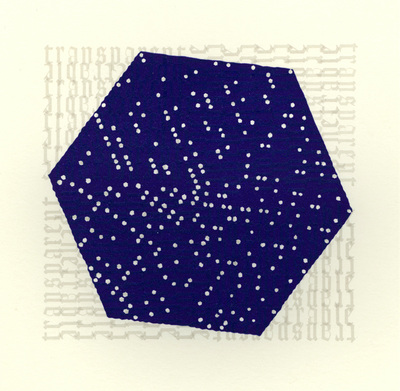
{2, 3, 5, 7, . . . 1789, 1801} and {2, 3, 5, 7, . . . 2203, 2207}
Meditations on the distribution of primes in the natural number sequence
These prints are maps of prime numbers. Mathematician Stanislaw Ulam discovered this phenomenon of these "strongly non-random patterns" by starting at a grid's center and assigning a natural number (1, 2, 3, . . . ) to each cell. As he spiraled outward, he marked the prime numbers so they were visually distinct from non-primes. A composition of diagonal lines emerged. This configuration is also referred to as Ulam's Cloth or Ulam's Rose. The hexagon to the right is Robert Sachs' spiral, made in the same fashion but using a hexagonal matrix (i.e. honeycomb pattern) rather than a typical grid.